Number Of Electrons In Aluminum

Learning Objectives

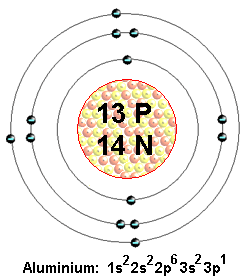
Name: Aluminum Symbol: Al Atomic Number: 13 Atomic Mass: 26.981539 amu Melting Point: 660.37 °C (933.52 K, 1220.666 °F) Boiling Point: 2467.0 °C (2740.15 K, 4472.6 °F) Number of Protons/Electrons: 13 Number of Neutrons: 14 Classification: Other Metals Crystal Structure: Cubic Density @ 293 K: 2.702 g/cm 3 Color: Silver British Spelling. In aluminum the configuration of the three outer electrons is such that in a few compounds (e.g., crystalline aluminum fluoride AlF 3 and aluminum chloride AlCl 3) the bare ion, Al 3+, formed by loss of these electrons, is known to occur. Aluminum is a Group IIIA element which means it has 3 electrons in it’s outer most shell, other wise known as each atom of aluminum has 3 valence electrons. Therefore aluminum atoms will lose 3 electrons so it takes on a + 3 charge when it becomes ion. This is an aluminium ion with a charge of +3. Having a +3 charge means aluminium having an atomic number 13 has lost three electrons. And since we know that in ordinary chemical reactions, electrons are the particles that are involved, this means.

By the end of this section, you will be able to:

Describe the classical free electron model of metals in terms of the concept electron number density
Explain the quantum free-electron model of metals in terms of Pauli’s exclusion principle
Calculate the energy levels and energy-level spacing of a free electron in a metal

Metals, such as copper and aluminum, are held together by bonds that are very different from those of molecules. Rather than sharing and exchanging electrons, a metal is essentially held together by a system of free electrons that wander throughout the solid. The simplest model of a metal is the free electron model. This model views electrons as a gas. We first consider the simple one-dimensional case in which electrons move freely along a line, such as through a very thin metal rod. The potential function (U(x)) for this case is a one-dimensional infinite square well where the walls of the well correspond to the edges of the rod. This model ignores the interactions between the electrons but respects the exclusion principle. For the special case of (T = 0 , K), (N) electrons fill up the energy levels, from lowest to highest, two at a time (spin up and spin down), until the highest energy level is filled. The highest energy filled is called the Fermi energy.

The one-dimensional free electron model can be improved by considering the three-dimensional case: electrons moving freely in a three-dimensional metal block. This system is modeled by a three-dimensional infinite square well. Determining the allowed energy states requires us to solve the time-independent Schrödinger equation

[-dfrac{h^2}{2m_c}left(dfrac{partial^2}{partial x^2} + dfrac{partial^2}{partial y^2} + dfrac{partial^2}{partial z^2}right) psi (x,y,z) = E psi (x,y,z), label{eq1}]

where we assume that the potential energy inside the box is zero and infinity otherwise. The allowed wave functions describing the electron’s quantum states can be written as

[psi(x,y,z) = left(sqrt{dfrac{2}{L_x}}sin dfrac{n_xpi x}{L_x}right) left(sqrt{dfrac{2}{L_y}}sin dfrac{n_ypi y}{L_y}right)left(sqrt{dfrac{2}{L_z}}sin dfrac{n_zpi z}{L_z}right), label{eq2}]

where (n_x, , n_y) and (n_z) are positive integers representing quantum numbers corresponding to the motion in the x-, y-, and z-directions, respectively, and (L_x), (L_y) and (L_z) are the dimensions of the box in those directions. Equation ref{eq2} is simply the product of three one-dimensional wave functions. The allowed energies of an electron in a cube ((L = L_x = L_y = L_z)) are

[E = dfrac{pi^2 hbar^2}{2mL^2} (n_1^2 + n_2^2 + n_3^2). label{eq3}]

Associated with each set of quantum numbers ((n_x, , n_y, , n_z)) are two quantum states, spin up and spin down. In a real material, the number of filled states is enormous. For example, in a cubic centimeter of metal, this number is on the order of (10^{22}). Counting how many particles are in which state is difficult work, which often requires the help of a powerful computer. The effort is worthwhile, however, because this information is often an effective way to check the model.

Example (PageIndex{1}): Energy of a Metal Cube

Consider a solid metal cube of edge length 2.0 cm.

What is the lowest energy level for an electron within the metal?
What is the spacing between this level and the next energy level?

Strategy

An electron in a metal can be modeled as a wave. The lowest energy corresponds to the largest wavelength and smallest quantum number: (n_x, , n_y, , n_z = (1,1,1)). Equation ref{eq3} supplies this “ground state” energy value. Since the energy of the electron increases with the quantum number, the next highest level involves the smallest increase in the quantum numbers, or ((n_x, , n_y, , n_z) = (2,1,1), (1,2,1),) or ((1,1,2)).

Solution

The lowest energy level corresponds to the quantum numbers (n_x = n_y = n_z = 1). From Equation ref{eq3}, the energy of this level is

[begin{align*} E(1,1,1) &= dfrac{pi^2 h^2}{2m_eL^2} (1^2 + 1^2 + 1^2) nonumber [4pt] &= dfrac{3pi^2 (1.05 times 10^{-34} , J cdot s)^2}{2(9.11 times 10^{-31} kg)(2.00 times 10^{-2} m)^2} nonumber [4pt] &= 4.48 times 10^{-34} Jnonumber [4pt] &= 2.80 times 10^{-15} eV. nonumber end{align*}]

The next-higher energy level is reached by increasing any one of the three quantum numbers by 1. Hence, there are actually three quantum states with the same energy. Suppose we increase (n_x) by 1. Then the energy becomes

[begin{align*} E(2,1,1) &= dfrac{pi^2h^2}{2m_eL^2} (2^2 + 1^2 + 1^2) nonumber [4pt] &= dfrac{6pi^2(1.05 times 10^{-34} , J cdot s)^2}{2(9.11 times 10^{-31} kg)(2.00 times 10^{-2}m)^2} nonumber [4pt] &= 8.96 times 10^{-34} J nonumber [4pt] &= 5.60 times 10^{-15} eV.nonumber end{align*}]

The energy spacing between the lowest energy state and the next-highest energy state is therefore

[E(2,1,1) - E(1,1,1) = 2.80 times 10^{-15} eV. nonumber]

Significance

This is a very small energy difference. Compare this value to the average kinetic energy of a particle, (k_BT), where (k_B) is Boltzmann’s constant and (T) is the absolute temperature. The product (k_BT) is about 1000 times greater than the energy spacing.

Exercise (PageIndex{1})

What happens to the ground state energy of an electron if the dimensions of the solid increase?

Answer

It decreases.

Often, we are not interested in the total number of particles in all states, but rather the number of particles dN with energies in a narrow energy interval. This value can be expressed by

[begin{align} dN &= n(E)dE nonumber [4pt] &= g(E)dE cdot F nonumber end{align} nonumber]

where (n(E)) is the electron number density, or the number of electrons per unit volume; (g(E)) is the density of states, or the number of allowed quantum states per unit energy; (dE) is the size of the energy interval; and (F) is the Fermi factor. The Fermi factor is the probability that the state will be filled. For example, if (g(E)dE) is 100 available states, but (F) is only 5%, then the number of particles in this narrow energy interval is only five. Finding (g(E)) requires solving Schrödinger’s equation (in three dimensions) for the allowed energy levels (Equation ref{eq1}). The calculation is involved even for a crude model, but the result is simple:

[g(E) = dfrac{pi V}{2} left(dfrac{8m_e}{h^2} right)^{3/2} E^{1/2},]

where V is the volume of the solid, (m_e) is the mass of the electron, and E is the energy of the state. Notice that the density of states increases with the square root of the energy. More states are available at high energy than at low energy. This expression does not provide information of the density of the electrons in physical space, but rather the density of energy levels in “energy space.” For example, in our study of the atomic structure, we learned that the energy levels of a hydrogen atom are much more widely spaced for small energy values (near than ground state) than for larger values.

This equation tells us how many electron states are available in a three-dimensional metallic solid. However, it does not tell us how likely these states will be filled. Thus, we need to determine the Fermi factor, F. Consider the simple case of (T = 0 , K). From classical physics, we expect that all the electrons ((approx 10^{22} / cm^3)) would simply go into the ground state to achieve the lowest possible energy. However, this violates Pauli’s exclusion principle, which states that no two electrons can be in the same quantum state. Hence, when we begin filling the states with electrons, the states with lowest energy become occupied first, then states with progressively higher energies. The last electron we put in has the highest energy. This energy is the Fermi energy (E_F) of the free electron gas. A state with energy (E < E_F) is occupied by a single electron, and a state with energy (E > E_F) is unoccupied. To describe this in terms of a probability F(E) that a state of energy E is occupied, we write for (T = 0 , K):

[F(E) = 1 , (E < E_F)]

[F(E) = 0 , (E > E_F).]

The density of states, Fermi factor, and electron number density are plotted against energy in Figure (PageIndex{1}).

A few notes are in order. First, the electron number density (last row) distribution drops off sharply at the Fermi energy. According to the theory, this energy is given by

[E_F = dfrac{h^2}{8m_e} left(dfrac{3 , N}{pi V} right)^{2/3}. label{eq5}]

Fermi energies for selected materials are listed in Table (PageIndex{1}). Note also that only the graph Figure (PageIndex{1c}), which answers the question, “How many particles are found in the energy range?” is checked by experiment. The Fermi temperature or effective “temperature” of an electron at the Fermi energy is

[T_F = dfrac{E_F}{k_B}.]

Table (PageIndex{1}): Conduction Electron Densities and Fermi Energies for Some Metals
Element	Conduction Band Electron Density ((10^{28}m^{-3}))	Free-Electron Model Fermi Energy ((eV))
Al	18.1	11.7
Ba	3.15	3.64
Cu	8.47	7.00
Au	5.90	5.53
Fe	17.0	11.1
Ag	5.86	5.49

Example (PageIndex{2}): Fermi Energy of Silver

Metallic silver is an excellent conductor. It has (5.89 times 10^{28}) conduction electrons per cubic meter. (a) Calculate its Fermi energy. (b) Compare this energy to the thermal energy (k_BT) of the electrons at a room temperature of 300 K.

Solution

From Equation ref{eq5}, the Fermi energy is [begin{align} E_F &= dfrac{h^2}{2m_e}(3pi^2n_e)^{2/3} nonumber [4pt] &= dfrac{(1.05 times 10^{-34} J cdot s)^2}{2(9.11 times 10^{-31}kg)} times [(3pi^2 (5.89 times 10^{28}m^{-3})]^{2/3} nonumber [4pt] &= 8.79 times 10^{-19}J = 5.49 , eV. nonumber end{align} nonumber] This is a typical value of the Fermi energy for metals, as can be seen from Table (PageIndex{1}).
We can associate a Fermi temperature (T_F) with the Fermi energy by writing (k_BT_F = E_F). We then find for the Fermi temperature [begin{align} T_F &= dfrac{8.79 times 10^{-19}J}{1.38 times 10^{-23} J/K} nonumber [4pt] &= 6.37 times 10^6 K,nonumber end{align} nonumber] which is much higher than room temperature and also the typical melting point ((approx 10^3 , K)) of a metal. The ratio of the Fermi energy of silver to the room-temperature thermal energy is [dfrac{E_F}{k_BT} = dfrac{T_F}{T} approx 210.]

To visualize how the quantum states are filled, we might imagine pouring water slowly into a glass, such as that of Figure (PageIndex{2}). The first drops of water (the electrons) occupy the bottom of the glass (the states with lowest energy). As the level rises, states of higher and higher energy are occupied. Furthermore, since the glass has a wide opening and a narrow stem, more water occupies the top of the glass than the bottom. This reflects the fact that the density of states g(E) is proportional to (E^{1/2}), so there is a relatively large number of higher energy electrons in a free electron gas. Finally, the level to which the glass is filled corresponds to the Fermi energy.

Suppose that at (T = 0 , K), the number of conduction electrons per unit volume in our sample is (n_e). Since each field state has one electron, the number of filled states per unit volume is the same as the number of electrons per unit volume.

Contributors and Attributions

Samuel J. Ling (Truman State University), Jeff Sanny (Loyola Marymount University), and Bill Moebs with many contributing authors. This work is licensed by OpenStax University Physics under a Creative Commons Attribution License (by 4.0).

A. The number of valence electrons in aluminum is

1) 1e^– 2) 2e^– 3) 3e^–

B. The change in electrons for octet requires a

1) loss of 3e^–2) gain of 3e^–3) gain of 5e^–

C. The ionic charge of aluminum is

1) 3^– 2) 5^– 3) 3⁺

D. The symbol for the aluminum ion is

1) Al³⁺ 2) Al^3- 3) Al⁺

Show Answer

A. The number of valence electrons in aluminum is

3) 3e^–

B. The change in electrons for octet requires a

1) loss of 3e^–

C. The ionic charge of aluminum is

3) 3⁺

D. The symbol for the aluminum ion is

1) Al³⁺

A. Number of valence electrons in sulfur is

1) 4e^– 2) 6e^– 3) 8e^–

B. Change in electrons for octet requires a

1) loss of 2e^–2) gain of 2e^–3) gain of 4e^–

C. Ionic charge of sulfur is

1) 2+ 2) 2- 3) 4-

Show Answer

A. Number of valence electrons in sulfur is

2) 6e^–

B. Change in electrons for octet requires a

2) gain of 2e^–

C. Ionic charge of sulfur is

2) 2-

3. Select the correct formula for each of the following ionic compounds

A. Na⁺and S^2-

1) NaS 2) Na₂S 3) NaS₂

B. Al³⁺and Cl^–

1) AlCl₃ 2) AlCl 3) Al₃Cl

C. Mg²⁺ and N^3-

1) MgN 2) Mg₂N₃ 3) Mg₃N₂

Show Answer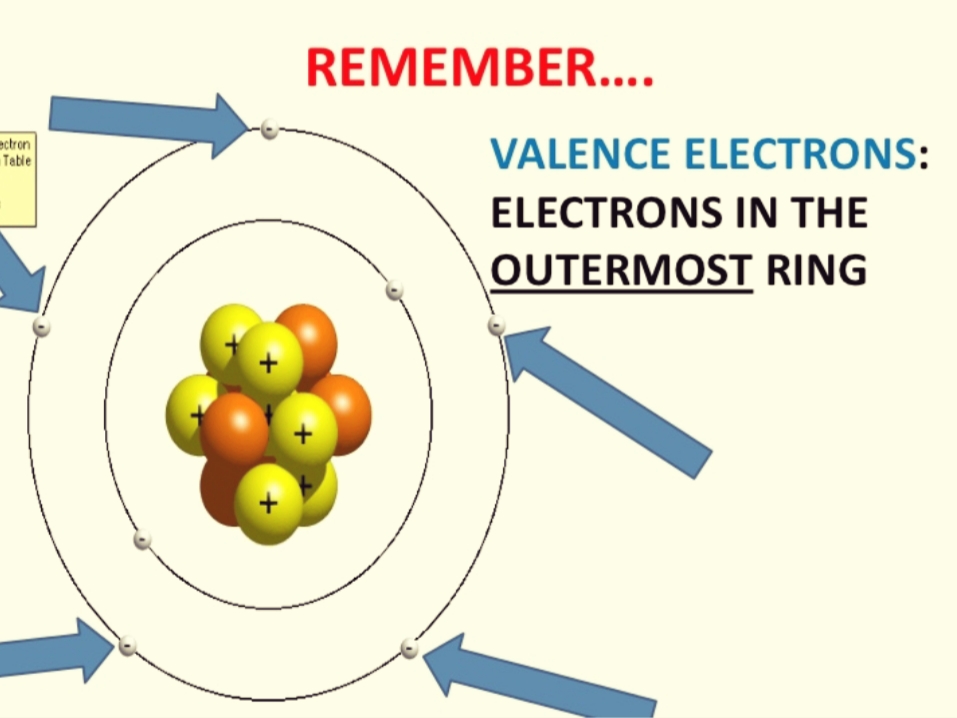

A. Na⁺and S^2-

2) Na₂S

check: 2Na⁺ + S^2- = 2(1+) + 1(2-) = 0

B. Al³⁺and Cl^–

1) AlCl₃

check: Al³⁺ + 3Cl^– = (3+) + 3(1-) = 0

C. Mg²⁺and N^3-

3) Mg₃N₂

check: 3Mg²⁺ +2N^3- = 3(2+) + 2(3-) = 0

4. Complete the names of the following ions:

Ba²⁺Al³⁺ K⁺

_________ __________ _________

N³^– O²^– F^–

_________ __________ _________

P³^– S²^– Cl^–

_________ __________ __________

Show Answer

Ba²⁺Al³⁺ K⁺

barium aluminum potassium

N³^– O²^– F^–

nitride oxide fluoride

P³^– S²^– Cl^–

phosphide sulfide chloride

5. Write the names of the following compounds:

1) CaO ___________

2) KBr ___________

3) Al₂O₃ ___________

4) MgCl₂ ___________

Show Answer

Write the names of the following compounds:

1) CaO calcium oxide

2) KBr potassium bromide

3) Al₂O₃ aluminum oxide

4) MgCl₂ magnesium chloride

6. Select the correct name for each.

A. Fe₂S₃

1) iron sulfide

2) iron(II) sulfide

3) iron (III) sulfide

B. CuO

1) copper oxide

2) copper(I) oxide

3) copper (II) oxide

Show Answer

Select the correct name for each.

A. Fe₂S₃

3) iron (III) sulfide Fe³⁺S^2-

B. CuO

3) copper (II) oxide Cu²⁺O^2-

7. The correct formula for each of the following is:

A. Copper (I) nitride

1) CuN 2) CuN₃ 3) Cu₃N

B. Lead (IV) oxide

1) PbO₂ 2) PbO 3) Pb₂O₄

Show Answer

The correct formula is

A. Copper (I) nitride

3) Cu₃N 3Cu⁺ + N^3-= 3(1+) + (3-) = 0

B. Lead (IV) oxide

1) PbO₂ Pb⁴⁺ + 2O^2-= (4+) +2(2-) = 0

8. Match each formula with the correct name:

A. MgS 1) magnesium sulfite

MgSO₃ 2) magnesium sulfate

MgSO₄ 3) magnesium sulfide

B. Ca(ClO₃)₂ 1) calcium chlorate

CaCl₂ 2) calcium chlorite

Ca(ClO₂)₂3) calcium chloride

Show Answer

Match each formula with the correct name:

A. MgS 3) magnesium sulfide

MgSO₃ 1) magnesium sulfite

MgSO₄ 2) magnesium sulfate

B. Ca(ClO₃)₂ 1) calcium chlorate

CaCl₂ 3) calcium chloride

Ca(ClO₂)₂2) calcium chlorite

9. Name each of the following compounds:

A. Mg(NO₃)₂

B. Cu(ClO₃)₂

C. PbO₂

D. Fe₂(SO₄)₃

E. Ba₃(PO₃⁾₂

Show Answer

Name each of the following compounds:

A. Mg(NO₃)₂magnesium nitrate

B. Cu(ClO₃)₂copper(II) chlorate

C. PbO₂lead (IV) oxide

D. Fe₂(SO₄)₃iron(III) sulfate

E. Ba₃(PO₃)₂barium phosphite

10. Write the correct formula for each:

A. potassium bromate

B. calcium carbonate

C. sodium phosphate

D. iron(III) oxide

E. iron (II) nitrite

Show Answer

Write the correct formula for each:

A. potassium bromate KBrO₃

B. calcium carbonate CaCO₃

C. sodium phosphate Na₃PO₄

D. iron(III) oxide Fe₂O₃

E. iron (II) nitrite Fe(NO₂)₂

11. Name the following compounds:

A. Ca₃(PO₄)₂

B. FeBr₃

C. Al₂S₃

D. Zn(NO₂)₂

E. NaHCO₃

Show Answer

Name the following compounds:

A. Ca₃(PO₄)₂Ca²⁺ PO₄³⁻calcium phosphate

B. FeBr₃Fe³⁺ Br⁻iron(III) bromide

C. Al₂S₃Al³⁺ S²⁻aluminum sulfide

D. Zn(NO₂)₂Zn²⁺ NO₂⁻zinc nitrite

E. NaHCO₃Na⁺ HCO₃⁻sodium hydrogen carbonate

12. Write the formulas for the following:

A. calcium nitrate

B. iron(II) hydroxide

C. aluminum carbonate

D. copper(II) bromide

E. lithium phosphate

Show Answer

Write the formulas for the following:

A. calcium nitrate Ca²⁺, NO₃⁻ Ca(NO₃)₂

B. iron(II) hydroxide Fe²⁺, OH⁻ Fe(OH)₂

C. aluminum carbonate Al³⁺, CO₃²⁻ Al₂(CO₃)₃

D. copper(II) bromide Cu²⁺, Br⁻ CuBr₂

E. lithium phosphate Li⁺, PO₄³⁻ Li₃PO₄

13. Match each formula with the correct name:

A. MgS 1) magnesium sulfite

MgSO₃ 2) magnesium sulfate

MgSO₄ 3) magnesium sulfide

B. Ca(ClO₃)₂ 1) calcium chlorate

CaCl₂ 2) calcium chlorite

Ca(ClO₂)₂3) calcium chloride

Show Answer

Match each formula with the correct name:

What is the total number of electrons in aluminum

A. MgS 3) magnesium sulfide

MgSO₃ 1) magnesium sulfite

MgSO₄ 2) magnesium sulfate

B. Ca(ClO₃)₂ 1) calcium chlorate

CaCl₂ 3) calcium chloride

Ca(ClO₂)₂2) calcium chlorite

14. Name each of the following compounds:

A. Mg(NO₃)₂

B. Cu(ClO₃)₂

C. PbO₂

D. Fe₂(SO₄)₃

E. Ba₃(PO₃⁾₂

Show Answer

Name each of the following compounds:

A. Mg(NO₃)₂magnesium nitrate

B. Cu(ClO₃)₂copper(II) chlorate

C. PbO₂lead (IV) oxide

D. Fe₂(SO₄)₃iron(III) sulfate

E. Ba₃(PO₃)₂barium phosphite

15. Select the correct formula for each:

A. aluminum nitrate

1) AlNO₃2) Al(NO)₃ 3) Al(NO₃)₃

B. copper(II) nitrate

1) CuNO₃ 2) Cu(NO₃)₂ 3) Cu₂(NO₃)

C. iron (III) hydroxide

1) FeOH 2) FeOH₃3) Fe(OH)₃

D. tin(IV) hydroxide

1) Sn(OH)₄ 2) Sn(OH)₂ 3) Sn₄(OH)

Show Answer

Select the correct formula for each:

A. aluminum nitrate

3) Al(NO₃)₃

B. copper(II) nitrate

2) Cu(NO₃)₂

C. iron(III) hydroxide

3) Fe(OH)₃

D. tin(IV) hydroxide

1) Sn(OH)₄

16. Write the correct formula for each:

A. potassium bromate

B. calcium carbonate

C. sodium phosphate

D. iron(III) oxide

E. iron (II) nitrite

Show Answer

Write the correct formula for each:

A. potassium bromate KBrO₃

B. calcium carbonate CaCO₃

C. sodium phosphate Na₃PO₄

D. iron(III) oxide Fe₂O₃

E. iron (II) nitrite Fe(NO₂)₂

17. Name the following compounds:

A. Ca₃(PO₄)₂

B. FeBr₃

C. Al₂S₃

D. Zn(NO₂)₂

E. NaHCO₃

Show Answer

Name the following compounds:

A. Ca₃(PO₄)₂Ca²⁺ PO₄³⁻calcium phosphate

B. FeBr₃Fe³⁺ Br⁻iron(III) bromide

C. Al₂S₃Al³⁺ S²⁻aluminum sulfide

D. Zn(NO₂)₂Zn²⁺ NO₂⁻zinc nitrite

E. NaHCO₃Na⁺ HCO₃⁻sodium hydrogen

18. Write the formulas for the following:

A. calcium nitrate

B. iron(II) hydroxide

C. aluminum carbonate

D. copper(II) bromide

E. lithium phosphate

Show Answer

Write the formulas for the following:

What Are The Number Of Electrons In Aluminum

A. calcium nitrate Ca²⁺, NO₃⁻ Ca(NO₃)₂

Number Of Valence Electrons In Aluminum

B. iron(II) hydroxide Fe²⁺, OH⁻ Fe(OH)₂

C. aluminum carbonate Al³⁺, CO₃²⁻ Al₂(CO₃)₃

Number Of Electrons In Aluminum Wire

D. copper(II) bromide Cu²⁺, Br⁻ CuBr₂

Number Of Electrons In Aluminum Oxide

E. lithium phosphate Li⁺, PO₄³⁻ Li₃PO₄